各种圆与圆相交阴影面积

前言

好久没有使用mathematica了，今天正好看到一个求圆相交情况下阴影的面积的问题（然后没有能够手算出来是多少），就想使用mathematica来计算一下结果，算完之后正好把其他的几种情况也都算了一下，放在这里。

好久没用mathematica，发现还是一样好用。

问题合集

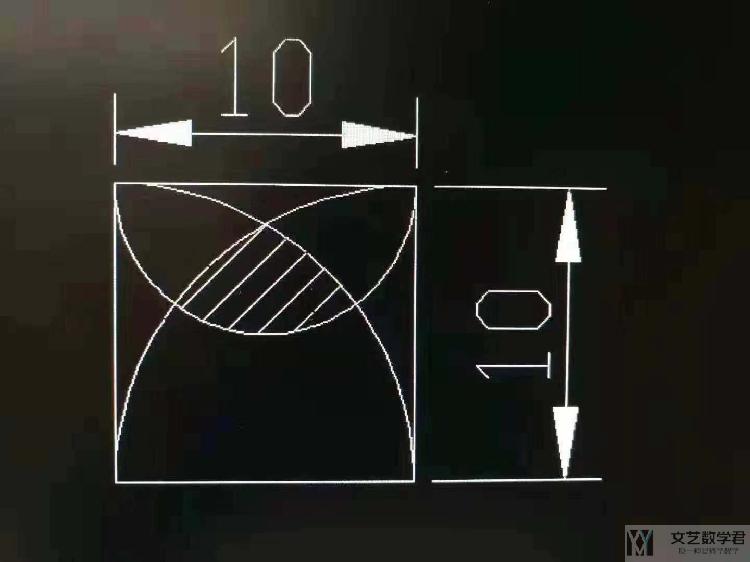
问题一：三圆相交，求阴影面积

要求下图的阴影面积，我尝试了好几次没试出来，<( )>，就用mathematica来计算了。

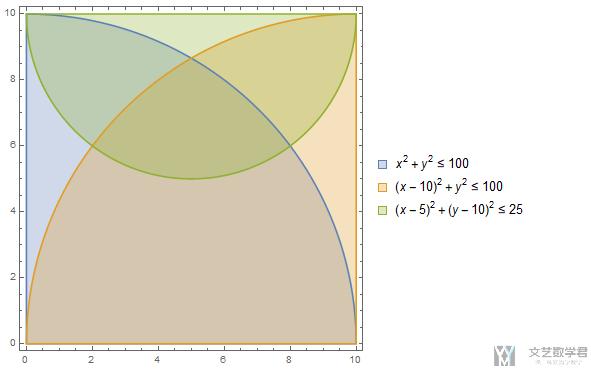
我们首先先把图像画出来看一下：

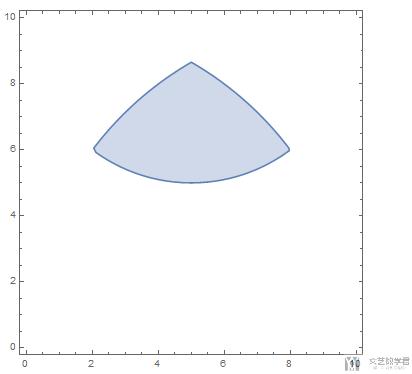
我们再把阴影部分的形状画出来：

下面我们来计算该阴影部分的面积，我们直接使用积分进行计算：

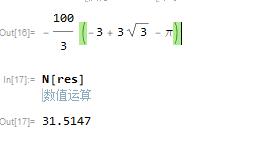
上面图片压缩了有点看不清，这里再说一下，解析解是：

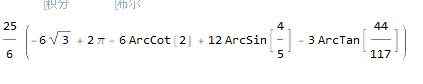
到这里把第一个的阴影部分面积计算完毕了，大家如果有知道如何手算出解析解的，可以在下面留言。

问题二：三圆两两相交圆心，求阴影面积

这是第二个问题，求下面三个圆相交部分的阴影面积：

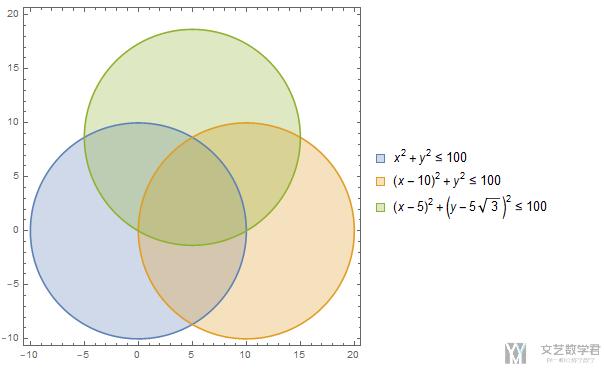
求面积的方法还是和上面一样的方法，就不详细的截图了，看一下阴影部分的形状：

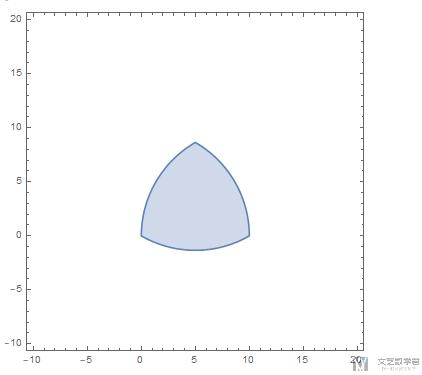
下面就直接求阴影部分的面积：

问题三：四圆相交，求阴影面积

跟上面都是一样的步骤，这里就更加简略一些了，首先画出图像：

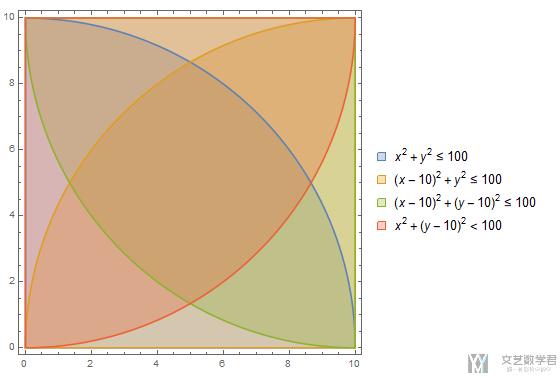
接着画出相交部分的面积：

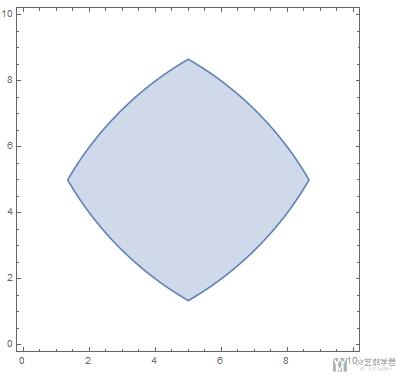
最后求出上面这部分的面积：

结语

怎么说呢，其实写这一篇文章就是想写写关于mathematica的东西吧，好久没有写了吗，以前有一段时间特别喜欢用，现在开始用的越来越少了。

不知道怎么说，真的很神奇。总之就记录下来吧。

有一篇类似的文章，是求圆柱与球相交的体积的，与这个类似，我把链接放在这里：

圆柱与球相交体体积计算及扩展问题